I was helping a friend learn L’Hôpital’s Rule. This is a Freshman Calculus thing. (A different one from last week, it happens. Folks are going back to school, I suppose.) The friend asked me a point I thought shouldn’t come up. I’m certain it won’t come up in the exam my friend was worried about, but I couldn’t swear it wouldn’t happen at all. So this is mostly a note to myself to think it over and figure out whether the trouble could come up. And also so this won’t be my most accessible post; I’m sorry for that, for folks who aren’t calculus-familiar.
L’Hôpital’s Rule is a way of evaluating the limit of one function divided by another, of f(x) divided by g(x). If the limit of 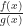 has either the form of
has either the form of 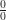 or
or  then you’re not stuck. You can take the first derivative of the numerator and the denominator separately. The limit of
then you’re not stuck. You can take the first derivative of the numerator and the denominator separately. The limit of 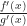 if it exists will be the same value.
if it exists will be the same value.
But it’s possible to have to do this several times over. I used the example of finding the limit, as x grows infinitely large, where f(x) = x2 and g(x) = ex.  goes to
goes to  as x grows infinitely large. The first derivatives,
as x grows infinitely large. The first derivatives, 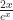 , also go to
, also go to  . You have to repeat the process again, taking the first derivatives of numerator and denominator again.
. You have to repeat the process again, taking the first derivatives of numerator and denominator again. 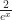 finally goes to 0 as x gets infinitely large. You might have to do this a bunch of times. If f(x) were x7 and g(x) again ex you’d properly need to do this seven times over. With experience you figure out you can skip some steps. Of course students don’t have the experience to know they can skip ahead to the punch line there, but that’s what the practice in homework is for.
finally goes to 0 as x gets infinitely large. You might have to do this a bunch of times. If f(x) were x7 and g(x) again ex you’d properly need to do this seven times over. With experience you figure out you can skip some steps. Of course students don’t have the experience to know they can skip ahead to the punch line there, but that’s what the practice in homework is for.
Anyway, my friend asked whether it’s possible to get a pattern that always ends up with 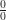 or
or  and never breaks out of this. And that’s what’s got me stuck. I can think of a few patterns that would. Start out, for example, with f(x) = e3x and g(x) = e2x. Properly speaking, that would never end. You’d get an infinity-over-infinity pattern every derivative you took. Similarly, if you started with
and never breaks out of this. And that’s what’s got me stuck. I can think of a few patterns that would. Start out, for example, with f(x) = e3x and g(x) = e2x. Properly speaking, that would never end. You’d get an infinity-over-infinity pattern every derivative you took. Similarly, if you started with 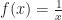 and
and  you’d never come to an end. As x got infinitely large both f(x) and g(x) would go to zero and all their derivatives would be zero over and over and over and over again.
you’d never come to an end. As x got infinitely large both f(x) and g(x) would go to zero and all their derivatives would be zero over and over and over and over again.
But those are special cases. Anyone looking at what they were doing instead of just calculating would look at, say, 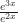 and realize that’s the same as
and realize that’s the same as 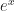 which falls out of the L’Hôpital’s Rule formulas. Or
which falls out of the L’Hôpital’s Rule formulas. Or  would be the same as
would be the same as 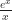 which is an infinity-over-infinity form. But it takes only one derivative to break out of the infinity-over-infinity pattern.
which is an infinity-over-infinity form. But it takes only one derivative to break out of the infinity-over-infinity pattern.
So I can construct examples that never break out of a zero-over-zero or an infinity-over-infinity pattern if you calculate without thinking. And calculating without thinking is a common problem students have. Arguably it’s the biggest problem mathematics students have. But what I wonder is, are there ratios that end up in an endless zero-over-zero or infinity-over-infinity pattern even if you do think it out?
And thus this note; I’d like to nag myself into thinking about that.
!['And ... My batting average of predicting the [unborn child's] sex is 97% accurate!'](https://nebusresearch.files.wordpress.com/2013/02/rex-morgan-7-february-2013.gif?w=840)

